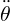
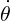
Physics 238—Intermediate Physics Lab
Homework Assignment #4
Due Monday, April 21, 2025, 1:15 p.m.
In this assignment, you will explore the relation between the width of a resonance peak and the lifetime of a damped oscillator.
To start, consider the decaying oscillations of a damped torsional oscillator. The differential equation for a damped, torsional oscillator of torsional constant κ and rotational inertia I is
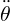| | = -ω02θ - γ |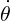
where ω0 = 2πf0 = 
is the natural (undamped) frequency of the oscillator, and γ is a
term proportional to the drag, with units of rads-1. If released from rest from an initial angle θ0,
the resulting motion is a damped oscillation:
| θ(t) | = θ0 e-γt∕2 cos(ω vt + ϕ) . |
For this assignment, the key observation is to note that the amplitude decays exponentially:
| A | = θ0e-γt∕2 = θ 0e-t∕(2τ) |
where τ = 1∕γ is the time constant, or lifetime, associated with the motion. Recall that the energy of a simple harmonic oscillator is proportional to the amplitude squared, so we can also describe the decay in terms of energy:
| A2 | = E = E 0e-γt = E 0e-t∕τ | (1) |
If periodic forcing at frequency ωd is added, the new differential equation is
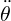 | = -ω02θ - γ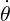 + α
0 sin |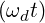
where α0 =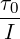 
is the amplitude of the driving. After an initial transient, the steady state
motion is given by a sinusoidal oscillation with an amplitude θ0 given by
| θ0 | =  |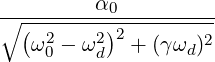
As with the decaying oscillation, the energy is related to the amplitude squared
| θ02 | = 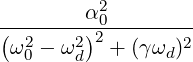 | (2) |
In this assignment, you will explore the relation between the lifetime τ of the damped oscillations and the width of the peak in Eq. 2. Throughout, it will be simpler to leave things in terms of ω and γ (or τ), rather than using f and Q.
Hint: Assume that the damping is small, so that γ ≪ ω0 (or equivalently Q ≫ 1) so that you may drop higher order terms in γ∕ω0, and use the binomial expansion when appropriate.
Assignment 1: What frequency ωd gives the maximum for θ02 in Eq. 2? Call that frequency ωp. What is the magnitude of θ02 at that frequency?
Assignment 2: What are the two frequencies that give an amplitude in Eq. 2 equal to one half of that maximum? Only keep terms up to the lowest order in γ∕ω0.
Assignment 3: Define the full width at half maximum—FWHM—as the difference between the two frequencies you found in assignment 2. Find the relation between the FWHM and τ in Eq. 1.
Assignment 4: Test your prediction for your mechanical resonance curve for the torsional
oscillator. Note that since you measured amplitude θ and not θ2, you will measure the FWHM by
noting the frequencies at which your curve is at a height of 
of the maximum. Note too that
you measured linear frequencies f, not angular frequencies ω, so you will need to manually
handle the factor of 2π.
Assignment 5: Test your prediction for your electrical resonance curve for the RLC oscillator.
Again, you will actually need to measure the frequencies at which your curve is at a height of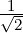 
of the maximum, and compensate for factors of 2π.
Your result should illustrate a quite general relation between the width of a resonance curve and the lifetime of the corresponding state.